Flow Rate of Fluid in Pipe Calculator

Pressure Change (\( \Delta P \)):

Pipe Radius (\( r \)):

Fluid Viscosity (\( \eta \)):

Length of Pipe (\( L \)):

1. What is Flow Rate of Fluid in Pipe Calculator?

Definition: This calculator computes the flow rate (\( Q \)) of a fluid in a pipe, based on the pressure change (\( \Delta P \)), pipe radius (\( r \)), fluid viscosity (\( \eta \)), and pipe length (\( L \)).

Purpose: It assists engineers in determining the volumetric flow rate of a fluid through a pipe, which is crucial for designing piping systems, HVAC systems, and fluid transport applications.

2. How Does the Calculator Work?

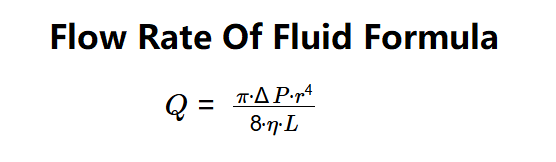
The calculator uses the Hagen-Poiseuille equation:

\( Q = \frac{\pi \cdot \Delta P \cdot r^4}{8 \cdot \eta \cdot L} \)

Where:

\( \Delta P \): Pressure change (in Pa, psi, kPa, or bar);
\( r \): Pipe radius (in m, mm, cm, in, ft, or yd);
\( \eta \): Fluid viscosity (in kg/(m·s), Pa·s, or lb/(ft·s));
\( L \): Length of the pipe (in m, mm, cm, in, ft, or yd);
\( Q \): Flow rate (in m³/s, in³/s, cm³/s, L/s, gal/s, gal/min, ft³/s, ft³/h, or m³/h);
Results are displayed with 3 decimal places (or scientific notation if less than 0.001).

Steps:

Enter the pressure change (\( \Delta P \)) and select the unit (Pa, psi, kPa, bar).
Enter the pipe radius (\( r \)) and select the unit (m, mm, cm, in, ft, yd).
Enter the fluid viscosity (\( \eta \)) and select the unit (kg/(m·s), Pa·s, lb/(ft·s)).
Enter the pipe length (\( L \)) and select the unit (m, mm, cm, in, ft, yd).
Click "Calculate" to compute the flow rate.
Change the result unit dropdown to convert the flow rate to a different unit.

3. Importance of Flow Rate Calculation

Calculating the flow rate of fluid in a pipe is crucial for:

Fluid Dynamics: Understanding how fluids move through pipes in various engineering applications.
Piping Design: Ensuring pipes are appropriately sized for desired flow rates in plumbing, HVAC, or industrial systems.
Energy Efficiency: Optimizing pressure and pipe dimensions to minimize energy losses due to friction.

4. Using the Calculator

Example 1: Calculate the flow rate with \( \Delta P = 1000 \, \text{Pa} \), \( r = 0.01 \, \text{m} \), \( \eta = 0.001 \, \text{kg/(m·s)} \), \( L = 2 \, \text{m} \), result in m³/s:

Pressure Change: 1000 Pa;
Pipe Radius: 0.01 m;
Fluid Viscosity: 0.001 kg/(m·s);
Pipe Length: 2 m;
\( Q = \frac{\pi \cdot 1000 \cdot (0.01)^4}{8 \cdot 0.001 \cdot 2} \);
\( (0.01)^4 = 0.00000001 \), so \( Q = \frac{3.1416 \cdot 1000 \cdot 0.00000001}{0.016} \approx \frac{0.000031416}{0.016} \approx 0.002 \, \text{m³/s} \);
Result: Flow Rate = 0.002 m³/s.

Example 2: Calculate the flow rate with \( \Delta P = 1 \, \text{psi} \), \( r = 1 \, \text{in} \), \( \eta = 0.001 \, \text{Pa·s} \), \( L = 1 \, \text{ft} \), result in gal/min:

Pressure Change: 1 psi = \( 1 \times 6894.76 = 6894.76 \, \text{Pa} \);
Pipe Radius: 1 in = \( 1 \times 0.0254 = 0.0254 \, \text{m} \);
Fluid Viscosity: 0.001 Pa·s = 0.001 kg/(m·s);
Pipe Length: 1 ft = \( 1 \times 0.3048 = 0.3048 \, \text{m} \);
\( Q = \frac{\pi \cdot 6894.76 \cdot (0.0254)^4}{8 \cdot 0.001 \cdot 0.3048} \);
\( (0.0254)^4 \approx 0.000000416 \), so \( Q \approx \frac{3.1416 \cdot 6894.76 \cdot 0.000000416}{0.0024384} \approx \frac{0.009}{0.0024384} \approx 3.692 \, \text{m³/s} \);
Convert to gal/min: \( 3.692 \times 15850.3 \approx 58517.408 \, \text{gal/min} \);
Result: Flow Rate = 58517.408 gal/min.

5. Frequently Asked Questions (FAQ)

Q: What is the Flow Rate of Fluid in a Pipe?
A: It is the volumetric rate at which a fluid flows through a pipe, determined by the pressure difference, pipe radius, fluid viscosity, and pipe length.

Q: Why is fluid viscosity important?
A: Fluid viscosity affects the resistance to flow; higher viscosity results in a lower flow rate for the same pressure difference and pipe dimensions.

Q: How does pipe radius affect the flow rate?
A: The flow rate is proportional to the fourth power of the radius, so small increases in radius lead to significant increases in flow rate.